Career Interest: Physics
As a result of reading in Language Arts and a couple of math projects, I grew a new interest in the 10th grade: Physics.
The Ferris Wheel project was about designing a Ferris Wheel. We had received a (fake) proposal from the (fake) “Greater Dayton Region” to boost tourism in Dayton. They saw that the SkyStar (a Ferris wheel) was very successful in Cincinnati. Our job was to design a Ferris wheel, make a model, and figure out the logistics behind the Ferris wheel, so that it could be commissioned as soon as possible. To figure out the logistics, we had to use a lot of math to effectively model the ride. We had to map the trajectory of the Ferris wheel, find equations to find the height of the rider at any point in time, and so forth. We were able to use the “Unit Circle Definition” of Trigonometry since our Ferris wheels were, in essence, circles. Our idea to create a Ferris Wheel was as followed: A spinning big wheel with spinning smaller wheels on it. The carts would be on the small spinning wheel.
This description may seem like a lot of math, but the physics comes in when we had to calculate the speed of the Ferris wheel. v = d/t: This read out “velocity is the distance traveled divided by the time it took to travel that distance”. It is a metric for how fast something is moving through space. It is easy to measure the time variable, but it became very hard to calculate the length traveled since the trajectory of our Ferris wheel was unique. We had the equation of the trajectory, but we had to research a way to find the length of the line formed by our trajectory. This proved to be very difficult, since it required us to use elliptical integrals a concept I was not familiar with yet. After reading up on them, we successfully computed the arc length of our trajectory. From there, we calculated the average velocity of the Ferris wheel. This research and analysis introduced me to the mathematical side of Physics. The proposal below shows the equations and work done.
Every other quarter in Language Arts, we were required to read a book on our own time. This book had to be at a level that was challenging, and at least 300 words. After researching books to read, one book instantly caught my attention: A Brief History of Time by Stephen Hawking. I had heard a lot about the book, and instantly checked it out at the library. As I read the reviews online, I was skeptical about this book because it seemed like many readers were confused after reading the book. Ignoring their advice, I began reading the book and was instantly hooked.
A Brief History of Time is a book discussing everything about physics. Stephen Hawking talks first about classical physics: dealing with the forces of nature observed by old physicists. He then talks about modern physicists, like Albert Einstein, who improved upon or disproved some of classical physics (space and time). He then goes on to talk about his own research in Black Holes, and what information we get from observing them. Finally, he talks about time, and why it is the way it is. He does all of this using barely any technicality; the only equation he presents in the whole book is E=mc*c – The classic physics equation derived by Albert Einstein which shows that energy is proportional to mass. As one can tell, there is a lot of theory discussed in the book. After reading the book, I had a very good foundation in physics. Reading the book was not satisfactory, though. Some of the concepts in the book felt ambiguous (possibly because Hawking refrained from using technical information), so I used online resources to help me. This furthered my knowledge in the subject, and helped me greatly in the next endeavor in math.
The Tennis Ball Project was completed in Math. This project required us to create a solution to a problem common to most classrooms in the country: the noise produced by dragging a chair across the floor. Our school currently has installed tennis balls on each of the chair legs. This seemed like an effective solution at first, but the users soon realized the drawbacks: The felt on the tennis balls picks up dirt off the floor; the tennis ball gets very dirty. The tennis balls also make it hard to push the chair into the table. People leave chairs not pushed in – out of pure frustration. In our solution, we had to address two problems and effectively solve them. We then had to present how our prototype was better than the tennis balls. Our approach to find the optimal solution was to do heavy testing. Each aspect of our final prototype was tested and made sure that it was the best.
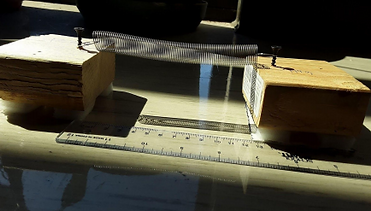
We found many materials to test: Felt, Satin, Leather, and Flannel. This would be the material that would be in constant contact with the floor. To test which material was the best, we devised a way to test the coefficient of friction. The coefficient of friction is a metric for the friction between two surfaces; it lets one compare the friction of two materials. The equation for the coefficient of friction is: m=Ff/Fn , where Ff is the frictional force, and Fn is the normal force. To test the frictional force, we set up an experiment. Two blocks of wood had nails attached to the sides so that they would be perpendicular to the ground. A spring was hooked to both nails. One of the blocks was meant to be stationary while the other was mobile. Under the block that was mobile, we put the material that we wanted to test; moving the block would now test the material’s friction against the ground. For each material we recorded how far we could extend the spring before the block would snap back. The normal force is essentially the force the earth beneath us exerts to stop us from going through the floor. With this data compiled in an excel sheet, we were able to analyze the coefficients of friction for each of the material.
This project especially improved my knowledge of forces. I learned the different kinds of forces that act upon an object and how to calculate them. My understanding of laws in physics also increased. This was all done primarily through research.

These projects throughout my Sophomore year have guided me in what I am going to do in the future. The book report I did on Stephen Hawking’s book introduced me to some physics content. I was later able to apply this knowledge to the projects in math. As a result of these experiences, I decided to choose physics as my elective next year. I hope to learn the content more rigorously next year. I think this year has prepared me well to succeed in that class, and any career involving physics.